Probability Lab
Probability Lab offers a practical way to think about options without the complicated mathematics.
This page introduces the following concepts:
Probability Distribution (PD)
The first concept to understand is the probability distribution (PD), which is a fancy way to say that all possible future outcomes have a chance or likelihood or probability of coming true. The PD tells us exactly what the chances are for certain outcomes. For example:
What is the probability that the daily high temperature in Hong Kong will be between 21.00 and 22.00 Celsius on November 22 next year?
We can take the temperature readings for November 22 for the last hundred years. Draw a horizontal line and mark it with 16 to 30 degrees and count how many readings fall into each one degree interval. The number of readings in each interval is the % probability that the temperature will be in that interval on November 22, assuming that the future will be like the past. It works out that way because we took 100 readings. Otherwise you must multiply by 100 and divide by the number of data points to get the percentages. In order to achieve greater accuracy we would need more points, so we could use data for November 20 through 24.
Let us draw a horizontal line spanning each one degree segment at the height corresponding to the number of data points in that segment. If we used data from November 20 through 24 we would get more data and greater accuracy but would need to multiply by 100 and divide by 500.
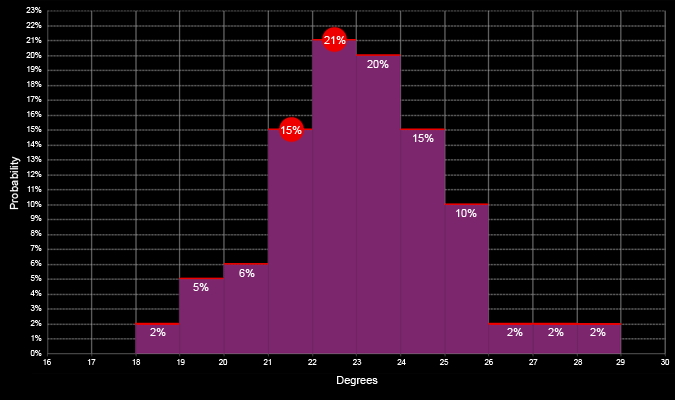
These horizontal lines compose a graph of our PD. They indicate the percentage likelihood that the temperature will be in any one interval. If we want to know the probability that the temperature will be below a certain level, we must add up all the probabilities in the segments below that level. In the same way we add up all the probabilities above the level if we want to know the probability of a higher temperature.
Accordingly, the graph indicates the probability for the temperature to be between 21 and 22 Celsius is 15% and the probability that it will be anywhere under 22 degrees is 2+5+6+15=28% and above 22 degrees is 100-28=72%.
Please note that the sum of the probabilities in all segments must add up to 1.00, i.e. there is a 100% chance that there will be some temperature in Hong Kong on that date.
If we had more data we could make our PD more precise by making the intervals narrower, and as we narrowed the intervals the horizontal lines would shrink to points forming a smooth bell shaped curve.
Stock Prices
Just the same way as future temperature ranges can be assigned probabilities, so can ranges of future stock prices or commodities or currencies. However, while temperature seems to follow the same pattern year after year, that is not true for stock prices which are more influenced by fundamental factors and human judgment.
So the answer to the question, "What is the probability that the price of ABC will be between 21.00 and 22.00 on November 22?" has to be more of an informed guess than the temperature in Hong Kong.
The information we have to work with is the current stock price, how it has moved in the past and fundamental data about the prospects of the company, the industry, the economy, currency, international trade and political considerations and so on, that may influence people's thinking about the stock price.
Forecasting the future stock price is an imprecise process. Forecasting the PD of future stock prices seems to allow more flexibility, or at least we become more aware of the probabilistic nature of the process. The more information and insight we have the more likely we are to get it right.
Options and How Their Prices Imply a PD
The prices of put and call options on a stock are determined by the PD but the interesting fact is that we can reverse engineer the process. Namely, given the prices of options, a PD implied by those prices can easily be derived. If you are interested in learning how to calculate the PD implied by options prices, here is a method you can follow.
Assume that stock XYZ is trading around $500 per share. What is the percentage probability that the price will be between 510 and 515 at the time the option expires about a month from now? Assume the 510 call trades at $6.45 and the 515 call trades at $4.40. You can buy the 510 call and sell the 515 call and pay $2.05.
- If at expiration time the stock is under 510, you lose $2.05
- If it is between 510 and 515, your gain is the average of your loss at 510 of $2.05 and your gain
at 515 of $2.95 or $0.45 - If it is above 515, you make $2.95
Further assume that we previously calculated that the probability for the stock to be below 510 is 56% or 0.56.*
Provided that options are "fairly" priced, i.e. there is no profit or loss that can be made if the market's PD is correct, then 0.56*-2.05+X*0.45+Y*2.95=0 where X=the probability that the stock will be between 510 and 515 and Y= the probability that it will be above 515.
Since all possible prices occurring have a probability of 100%, then 0.56+X+Y=1.00 gives us 0.06 for X and 0.38 for Y.
*To calculate an entire PD you need to start at the lowest strike and you need to take a guess as to the probability below that price. That will be a small number, so that you will not make too great an error.
If you've read this far then you will also be interested to know how you can derive the price of any call or put from the PD.
For a call you can take the stock price in the middle of each segment above the strike price, subtract the strike price and multiply the result by the probability of the price ending up in that segment. For the tail end you need to take a guess at the small probability and use a price about 20% higher than the high strike. Summing all the results gives you the call price.
For puts you can take the stock price in the middle of each interval below the strike, subtract it from the strike and multiply by the probability. For the last segment, between zero and the lowest strike I would use 2/3 of the lowest strike and guess the probability. Again, add all the results together to get the price of the put.
Some may say that these are all very sloppy approximations. Yes, that is the nature of predicting prices; they are sloppy and there is no point in pretending otherwise. Everybody is guessing. Nobody knows. Computer geeks with complex models appear to the uninitiated to be doing very precise calculations, but the fact is that nobody knows the probabilities and your educated guess based on your understanding of the situation may be better than theirs based on statistics of past history.
Note that we are ignoring interest effects in this discussion. We are also adjusting for the fact that options may be exercised early which makes them more valuable. When calculating the whole PD, this extra value needs to be accounted for but it is only significant for deep-in-the-money options. By using calls to calculate the PD for high prices and using puts to calculate the PD for low prices, you can avoid the issue.
The PD as Implied by the Market and Your Opinion
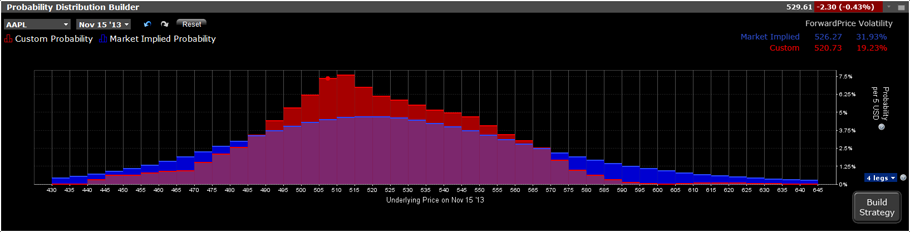
Given that puts and calls on most stocks are traded in the option markets, we can calculate the PD for those stocks as implied by the prevailing option prices. I call this the "market's PD," as it is arrived at by the consensus of option buyers and sellers, even if many may be unaware of the implications.
The highest point on the graph of the market's implied PD curve tends to be close to the current stock price plus interest minus dividends, and as you go in either direction from there the probabilities diminish, first slowly, then more rapidly and then slowly again, approaching but never quite reaching zero. The Forward Price is the expected price at expiration as implied by the probability distribution.
Click the image above to view a larger version
The curve is almost symmetrical except that slightly higher prices have higher probability than slightly lower ones and much higher prices have lesser probability than near zero ones. That's because prices tend to fall faster than they rise and all organizations have some chance of some catastrophic event happening to them.
In the Probability Lab you can view the PD we calculate using option prices currently prevailing in the market for any stock or commodity on which options are listed. All you need to do is to enter the symbol.
The PD graph changes as option bids and offers change at the exchanges. You can now grab the horizontal bar in any interval and move it up or down if you think that the price ending up in that interval has a higher or lower probability than the consensus guess as expressed by the market. You will notice that as soon as you move any of the bars, all the other bars will simultaneously move, with the more distant bars moving in the opposite direction as all the probabilities must add up to 1.00. Also notice that the market's PD remains on the display in blue while yours is red and the reset button will wipe out all of your doodling.
The market tends to assume that all PDs are close to the statistical average of past outcomes unless a definitive corporate action, such as a merger or acquisition, is in the works. If you follow the market or the particulars of certain stocks, industries or commodities, you may not agree with that. From time to time you may have a different view of the likelihood of certain events and therefore how prices may evolve. This tool gives you the facility to illustrate, to graphically express that view and to trade on that view. If you do not have an opinion of the PD as being different than the market's then you should not do a trade because any trade you do has a zero expected profit (less transaction costs) under the market's PD. The sum of each possible outcome (profit or loss in each interval) multiplied by its associated probability is the statistically Expected Profit and under the market's PD, it equals zero for any trade. You can pick any actual trade and calculate the expected profit to prove that to yourself. Thus, any time you do a trade with an expectation of profit, you are taking a bet that the market's PD is wrong and yours is right. This is true whether you are aware of it or not, so you may as well be aware of what you are doing and sharpen your skills with this tool.
The Best Trades and Their Potential Consequences
Please go ahead and play with the PD by dragging the distribution bars below. We display combination trades that are likely to have favorable outcomes under your PD. You can specify if you would like to see the "optimal trades" that are a combination of up to two, three or four option legs. We will show you the three best combination trades along with the corresponding expected profit, Sharpe ratio, net debit or credit, percentage likelihood of profit, maximum profit and maximum loss and associated probabilities for each trade, given your PD, and the margin requirement.
The best trades are the ones with the highest Sharpe ratio, or the highest ratio of expected profit to variability of outcome. Please remember that the expected profit is defined as the sum of the profit or loss when multiplied by the associated probability, as defined by you, across all prices. On the bottom graph you will see your predicted profit or loss that would result from the trade and the associated probability, corresponding to each price point.
The interactive graph below is a crude simulation of our real-time Probability Lab application that is available to our customers. Similarly, the "best trades" are displayed for illustrative purposes only. Unlike in the actual application, they are not optimized for your distribution.
When you like a trade in our trading application, you may increase the quantity and submit the order.
Free Probability Lab for Non-Customer
In subsequent releases of this tool we'll address buy writes, rebalancing for delta, multi-expiration combination trades, rolling forward of expiring positions and further refinements of the Probability Lab.
Please play around with this interactive tool. As you do so, your understanding of options pricing and your so called "feel for the options market" will deepen.
The projections or other information generated by the Probability Lab tool regarding the likelihood of various investment outcomes are hypothetical in nature, do not reflect actual investment results and are not guarantees of future results. Please note that results may vary with use of the tool over time.